Electron. J. Diff. Eqns., Vol. 2004(2004), No. 125, pp. 1-8.
Existence of solutions to n-dimensional pendulum-like equations
Pablo Amster, Pablo L. De Napoli, Maria Cristina Mariani
Abstract:
We study the elliptic boundary-value problem
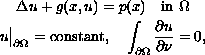
where
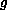 is
is
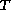 -periodic in
-periodic in
 ,
and
,
and
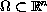 is a bounded domain.
We prove the existence of a solution under a condition on
the average of the forcing term
is a bounded domain.
We prove the existence of a solution under a condition on
the average of the forcing term
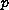 .
Also, we prove the existence of a compact interval
.
Also, we prove the existence of a compact interval
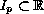 such that the problem is solvable for
such that the problem is solvable for
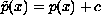 if and only if
if and only if
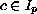 .
.
Submitted June 3, 2004. Published October 20, 2004.
Math Subject Classifications: 35J25, 35J65.
Key Words: Pendulum-like equations; boundary value problems;
topological methods.
Show me the
PDF file (213K),
TEX file, and other files for this article.
 |
Pablo Amster
Universidad de Buenos Aires
FCEyN - Departamento de Matemática
Ciudad Universitaria, Pabellóon I
(1428) Buenos Aires, Argentina
and Consejo Nacional de Investigaciones Científicas y
Técnicas (CONICET)
email: pamster@dm.uba.ar |
|---|
 |
Pablo L. De Nápoli
Universidad de Buenos Aires
FCEyN - Departamento de Matemáatica
Ciudad Universitaria, Pabellón I
(1428) Buenos Aires, Argentina
email: pdenapo@dm.uba.ar |
|---|
 |
María Cristina Mariani
Department of Mathematical Sciences
New Mexico State University
Las Cruces, NM 88003-0001, USA
email: mmariani@nmsu.edu |
|---|
Return to the EJDE web page
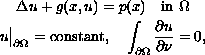
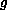 is
is
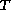 -periodic in
-periodic in
 ,
and
,
and
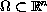 is a bounded domain.
We prove the existence of a solution under a condition on
the average of the forcing term
is a bounded domain.
We prove the existence of a solution under a condition on
the average of the forcing term
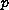 .
Also, we prove the existence of a compact interval
.
Also, we prove the existence of a compact interval
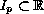 such that the problem is solvable for
such that the problem is solvable for
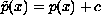 if and only if
if and only if
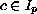 .
.


