Electron. J. Differential Equations, Vol. 2020 (2020), No. 44, pp. 1-15.
Multiple positive solutions for biharmonic equation of Kirchhoff type
involving concave-convex nonlinearities
Fengjuan Meng, Fubao Zhang, Yuanyuan Zhang
Abstract:
In this article, we study the multiplicity of positive solutions for the
biharmonic equation of Kirchhoff type involving concave-convex nonlinearities,
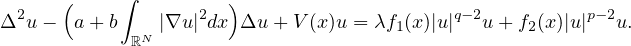
Using the Nehari manifold, Ekeland variational principle, and the theory
of Lagrange multipliers, we prove that there are at least two positive solutions,
one of which is a positive ground state solution.
Submitted March 11, 2019. Published May 19, 2020.
Math Subject Classifications: 35J35, 35J40, 35J91.
Key Words: Biharmonic equation; ground state solution; Nehari manifold;
concave-convex nonlinearity.
DOI: 10.58997/ejde.2020.44
Show me the PDF file (370 KB),
TEX file for this article.
 |
Fengjuan Meng
School of Mathematics and Physics
Jiangsu University of Technology
Changzhou 213001, China
email: fjmeng@jsut.edu.cn
|
|---|
 |
Fubao Zhang
School of Mathematics
Southeast University
Nanjing 210096, China
email: 101009933@seu.edu.cn
|
|---|
 |
Yuanyuan Zhang
School of Business
Jiangsu University of Technology
Changzhou 213001, China
email: zyylhh1227@163.com
|
|---|
Return to the EJDE web page