C. O. Alves, D. C. de Morais Filho, & M. A. S. Souto
Abstract:
We give a method for obtaining radially
symmetric solutions for the critical exponent problem
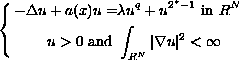
where, outside a ball centered at the origin, the non-negative function
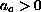 .
We remark that,
differently from the literature, we do not require any conditions on
.
We remark that,
differently from the literature, we do not require any conditions on
Submitted July 04, 1996. Published: August 30, 1996.
Math Subject Classification: 35A05, 35A15 and 35J20.
Key Words: Radial solutions, critical Sobolev exponents,
Palais-Smale condition, Mountain Pass Theorem.
Show me the PDF file (188 KB), TEX file, and other files for this article.