C. O. Alves
Abstract:
This article concerns with the problem
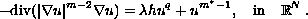
Using the Ekeland Variational Principle and the
Mountain Pass Theorem, we show the existence of
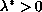 such that
there are at least two non-negative solutions for each
such that
there are at least two non-negative solutions for each
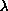 in
in
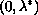 .
.
Submitted April 22, 1997. Published August 19, 1997.
Math Subject Classification: 35J20, 35J25.
Key Words: Mountain Pass Theorem, Ekeland Variational Principle.
Show me the PDF file (165 KB), TEX file, and other files for this article.