The analysis of Hill's operator
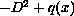 for
for
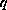 even and periodic
is extended from the real line to homogeneous trees
even and periodic
is extended from the real line to homogeneous trees
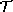 .
Generalizing the classical problem, a detailed analysis of Hill's equation and
its related operator theory on
.
Generalizing the classical problem, a detailed analysis of Hill's equation and
its related operator theory on
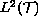 is provided.
The multipliers for this new version of Hill's equation
are identified and analyzed. An explicit description of the resolvent is given.
The spectrum is exactly described when the degree of the tree is greater than
two, in which case there are both spectral bands and eigenvalues.
Spectral projections are computed by means of an eigenfunction expansion.
Long time asymptotic expansions for the associated semigroup kernel
are also described.
A summation formula expresses the resolvent for a regular graph
as a function of the resolvent of its covering homogeneous tree
and the covering map. In the case of a finite regular graph,
a trace formula relates the spectrum of the Hill's operator
to the lengths of closed paths in the graph.
is provided.
The multipliers for this new version of Hill's equation
are identified and analyzed. An explicit description of the resolvent is given.
The spectrum is exactly described when the degree of the tree is greater than
two, in which case there are both spectral bands and eigenvalues.
Spectral projections are computed by means of an eigenfunction expansion.
Long time asymptotic expansions for the associated semigroup kernel
are also described.
A summation formula expresses the resolvent for a regular graph
as a function of the resolvent of its covering homogeneous tree
and the covering map. In the case of a finite regular graph,
a trace formula relates the spectrum of the Hill's operator
to the lengths of closed paths in the graph.