We investigate the dynamical effects of non-stationary boundaries on the stability of a quantum Hamiltonian system described by a periodic family
![$\{H(\gamma,t), t\in[0,\Gamma],\Gamma positive\}$](gifs/aa.gif) of Sturm-Liouville operators, a Schrodinger equation
of Sturm-Liouville operators, a Schrodinger equation
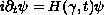 defined on
defined on 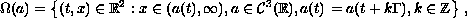
as well as boundary conditions at
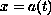 modeled by the
modeled by the
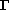 -periodic function
-periodic function
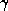 .
Employing extended Hilbert space methods, stability
conditions for the spectra of the evolution operators
.
Employing extended Hilbert space methods, stability
conditions for the spectra of the evolution operators
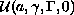 to the families
to the families
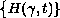 under perturbations induced by variations of boundary oscillations,
respectively conditions, are derived.
In particular, it is shown that the existence of a pure point finitely
degenerate realization
under perturbations induced by variations of boundary oscillations,
respectively conditions, are derived.
In particular, it is shown that the existence of a pure point finitely
degenerate realization
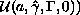 implies pure point
implies pure point
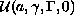 for all
for all
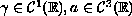 ,
whereas in case of infinitely degenerate
,
whereas in case of infinitely degenerate
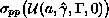 the existence of
the existence of
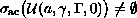 ,
respectively
,
respectively
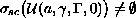 ,
is possible.
,
is possible.