We apply the Pohozaev identity to sub-domains of a tubular neighbourhood of a closed or broken curve in
 and establish uniqueness results for
the smooth solutions of the Dirichlet problem for
and establish uniqueness results for
the smooth solutions of the Dirichlet problem for 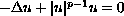 .
.We require the domain to be in
 with
with
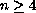 and with
and with
 .
.
Kewei Zhang (Macquarie University, Sydney, Australia)
Abstract:
We apply the Pohozaev identity to sub-domains of a tubular neighbourhood of
a closed or broken curve in
 and establish uniqueness results for
the smooth solutions of the Dirichlet problem for
and establish uniqueness results for
the smooth solutions of the Dirichlet problem for
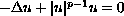 .
.
We require the domain to be in
 with
with
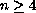 and with
and with
 .
.
Submitted May 12, 1999. Published September 15, 1999.
Math Subject Classifications: 35J65, 35B05, 58E05.
Key Words: semilinear elliptic equation, supercritical growth,
uniqueness, non-contractible domains, Pohozaev identity.
Show me the PDF file (134K), TEX file, and other files for this article.
 |
Kewei Zhang Department of Mathematics Macquarie University Sydney, Australia e-mail address: kewei@ics.mq.edu.au |
Return to the EJDE web page