Electron. J. Diff. Eqns., Vol. 1999(1999), No. 48, pp. 1-8.
On the smallness of the (possible) singular set in space for
3D Navier-Stokes equations
Zoran Grujic
Abstract:
We utilize
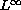 estimates on the complexified solutions of 3D
Navier-Stokes equations via a plurisubharmonic measure type maximum principle
to give a short proof of the fact that the Hausdorff dimension of the
(possible) singular set in space is less or equal 1 assuming chaotic,
Cantor set-like structure of the blow-up profile.
estimates on the complexified solutions of 3D
Navier-Stokes equations via a plurisubharmonic measure type maximum principle
to give a short proof of the fact that the Hausdorff dimension of the
(possible) singular set in space is less or equal 1 assuming chaotic,
Cantor set-like structure of the blow-up profile.
Submitted August 20, 1999. Published December 3, 1999.
Math Subject Classifications: 35Q30, 76D03.
Key Words: Navier-Stokes equations, singular
set, turbulence.
Show me the
PDF file (111K),
TEX file, and other files for this article.
Zoran Grujic
Department of Mathematics
University of Texas
Austin, TX 78712, USA
e-mail: grujic@math.utexas.edu
Return to the EJDE web page
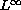 estimates on the complexified solutions of 3D
Navier-Stokes equations via a plurisubharmonic measure type maximum principle
to give a short proof of the fact that the Hausdorff dimension of the
(possible) singular set in space is less or equal 1 assuming chaotic,
Cantor set-like structure of the blow-up profile.
estimates on the complexified solutions of 3D
Navier-Stokes equations via a plurisubharmonic measure type maximum principle
to give a short proof of the fact that the Hausdorff dimension of the
(possible) singular set in space is less or equal 1 assuming chaotic,
Cantor set-like structure of the blow-up profile.