We study the existence of positive solutions for the following two nonlinear eigenvalue problems
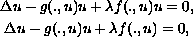
in a bounded regular domain in
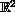 with
with
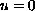 on the boundary. We assume that
on the boundary. We assume that
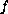 and
and
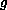 are
in Kato class of functions.
are
in Kato class of functions.
Nedra Belhaj Rhouma & Lamia Maatoug
Abstract:
We study the existence of positive solutions for the following
two nonlinear eigenvalue problems
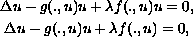
in a bounded regular domain in
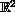 with
with
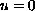 on the boundary. We assume that
on the boundary. We assume that
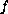 and
and
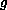 are
in Kato class of functions.
are
in Kato class of functions.
Submitted October 14, 2002. Published February 15, 2003.
Math Subject Classifications: 31A25, 31A35, 34B15, 34B27, 35J65
Key Words: Eigenvalue, Kato class, Green's function, superharmonic
function, Shauder fixed point theorem, maximum principle.
Show me the PDF file (234K), TEX file, and other files for this article.
 |
Nedra Belhaj Rhouma Institut Preparatoire aux Etudes d'ingenieurs de Tunis, 2 Rue Jawaherlal Nehru, 1008 Montfleury, Tunis, Tunisia. e-mail: Nedra.Belhajrhouma@ipeit.rnu.tn |
|---|---|
 |
Lamia Maatoug Departement de Mathematiques Faculte des Sciences de Tunis Campus universitaire 1060 Tunis, Tunisia e-mail: Lamia.Maatoug@ipeit.rnu.tn |
Return to the EJDE web page