In this article we consider a boundary-value problem for the equation
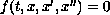 with mixed boundary conditions.
Assuming the existence of suitable barrier strips, and using
the monotone iterative method, we obtain the minimal and
maximal solutions.
with mixed boundary conditions.
Assuming the existence of suitable barrier strips, and using
the monotone iterative method, we obtain the minimal and
maximal solutions.
Myron K. Grammatikopoulos & Petio S. Kelevedjiev
Abstract:
In this article we consider a boundary-value problem for the
equation
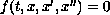 with mixed boundary conditions.
Assuming the existence of suitable barrier strips, and using
the monotone iterative method, we obtain the minimal and
maximal solutions.
with mixed boundary conditions.
Assuming the existence of suitable barrier strips, and using
the monotone iterative method, we obtain the minimal and
maximal solutions.
Submitted December 12, 2002. Published February 28, 2003.
Math Subject Classifications: 34B15.
Key Words: Boundary-value problems, minimal and maximal solutions,
monotone method, barrier strips.
Show me the PDF file (215K), TEX file, and other files for this article.
 |
Myron K. Grammatikopoulos Department of Mathematics University of Ioannina 451 10 Ioannina, Hellas, Greece e-mail: mgrammat@cc.uoi.gr |
|---|---|
 |
Petio S. Kelevedjiev Department of Mathematics Technical University of Sliven 8800 Sliven, Bulgaria e-mail: keleved@mailcity.com |
Return to the EJDE web page