Electron. J. Diff. Eqns., Vol. 2003(2003), No. 35, pp. 1-12.
Radial minimizer of a variant of the p-Ginzburg-Landau functional
Yutian Lei
Abstract:
We study the asymptotic behavior of the radial minimizer
of a variant of the p-Ginzburg-Landau functional when
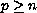 .
The location of the zeros and the uniqueness of the radial minimizer
are derived. We also prove the
.
The location of the zeros and the uniqueness of the radial minimizer
are derived. We also prove the
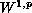 convergence of
the radial minimizer for this functional.
convergence of
the radial minimizer for this functional.
Submitted December 30, 2002. Published April 3, 2003.
Math Subject Classifications: 35J70, 49K20.
Key Words: Radial minimizer, variant of p-Ginzburg-Landau functional
Show me the
PDF file (215K),
TEX file, for this article.
Yutian Lei
Department of Mathematics
Nanjing Normal University
Nanjing, Jiangsu 210097, China
email: lythxl@163.com
Return to the EJDE web page
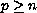 .
The location of the zeros and the uniqueness of the radial minimizer
are derived. We also prove the
.
The location of the zeros and the uniqueness of the radial minimizer
are derived. We also prove the
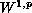 convergence of
the radial minimizer for this functional.
convergence of
the radial minimizer for this functional.