Electron. J. Diff. Eqns., Vol. 2003(2003), No. 42, pp. 1-10.

A discontinuous problem involving the p-Laplacian operator and critical exponent in $\mathbb{R}^N$

Claudianor Oliveira Alves & Ana Maria Bertone

Abstract:
Using convex analysis, we establish the existence of at least two nonnegative solutions for the quasilinear problem
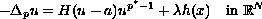
$$ -\Delta_{p}u=H(u-a)u^{p^*-1} +\lambda h(x)\quad\hbox{in }\mathbb{R}^N $$
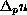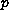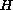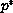where $\Delta_{p}u$ is the $p$-Laplacian operator, $H$ is the Heaviside function, $p^*$ is the Sobolev critical exponent, and 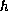$h$ is a positive function.

Submitted September 23, 2002. Published April 16, 2003.
Math Subject Classifications: 35A15, 35J60, 35H30.
Key Words: Variational methods, discontinuous nonlinearities, critical exponents.

Show me the PDF file (234K), TEX file, and other files for this article.

Claudianor Oliveira Alves
Universidade Federal de Campina Grande
Departamento de Matematica
58109-970 Campina Grande-PB, Brazil
email: coalves@dme.ufpb.br
Ana Maria Bertone
Universidade Federal da Paraiba
Departamento de Matematica
58059-900 Joao Pessoa-PB, Brazil
email: anita@mat.ufpb.br

Return to the EJDE web page