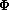 -Laplacian
when
-Laplacian
when
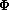 is a sup-multiplicative-like function
is a sup-multiplicative-like function
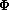 -Laplacian
when
-Laplacian
when
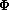 is a sup-multiplicative-like function
is a sup-multiplicative-like function
George L. Karakostas
Abstract:
The existence of triple positive solutions for a boundary-value
problem governed by the
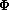 -Laplacian
is investigated, when
-Laplacian
is investigated, when
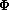 is a so-called sup-multiplicative-like function
(in a sense introduced in [22])
and the boundary conditions include nonlinear expressions at the
end points (as in [21, 28]). The Leggett-Williams fixed point
theorem in a cone is used. The results improve and generalize
known results given in [21].
is a so-called sup-multiplicative-like function
(in a sense introduced in [22])
and the boundary conditions include nonlinear expressions at the
end points (as in [21, 28]). The Leggett-Williams fixed point
theorem in a cone is used. The results improve and generalize
known results given in [21].
Submitted February 5, 2004. Published May 6, 2004.
Math Subject Classifications: 34B15, 34B18.
Key Words: Boundary value problems, positive solutions,
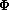 -Laplacian,
Leggett-Williams fixed point theorem.
-Laplacian,
Leggett-Williams fixed point theorem.
Show me the PDF file (241K), TEX file, and other files for this article.
 |
George L. Karakostas Department of Mathematics University of Ioannina, 451 10 Ioannina, Greece email: gkarako@cc.uoi.gr |
Return to the EJDE web page