Electron. J. Diff. Eqns., Vol. 2005(2005), No. 11, pp. 1-8.

Aleksandrov-type estimates for a parabolic Monge-Ampere equation

David Hartenstine

Abstract:
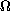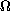A classical result of Aleksandrov allows us to estimate the size of a convex function $u$ at a point $x$ in a bounded domain $\Omega$ in terms of the distance from $x$ to the boundary of $\Omega$ if
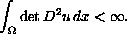
$$\int_{\Omega} \det D^{2}u \, dx less than \infty. $$
This estimate plays a prominent role in the existence and regularity theory of the Monge-Ampere equation. Jerison proved an extension of Aleksandrov's result that provides a similar estimate, in some cases for which this integral is infinite. Gutierrez and Huang proved a variant of the Aleksandrov estimate, relevant to solutions of a parabolic Monge-Ampere equation. In this paper, we prove Jerison-like extensions to this parabolic estimate.

Submitted January 12, 2005. Published January 27, 2005.
Math Subject Classifications: 35K55, 35B45, 35D99.
Key Words: Parabolic Monge-Ampere measure; pointwise estimates.

Show me the PDF file (217K), TEX file for this article.

David Hartenstine
Department of Mathematics
Western Washington University
516 High Street, Bond Hall 202
Bellingham, WA 98225-9063, USA
email: david.hartenstine@wwu.edu

Return to the EJDE web page