Electron. J. Diff. Eqns., Vol. 2005(2005), No. 46, pp. 1-8.
Rectifiability of solutions of the
one-dimensional p-Laplacian
Mervan Pasic
Abstract:
In the recent papers [8] and [10] a class of
Caratheodory functions
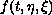 rapidly sign-changing
near the boundary point
rapidly sign-changing
near the boundary point
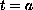 ,
has been constructed so that the
equation
,
has been constructed so that the
equation
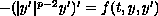 in
in
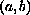 admits continuous
bounded solutions
admits continuous
bounded solutions
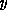 whose graphs
whose graphs
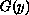 do not possess a finite
length. In this paper, the same class of functions
do not possess a finite
length. In this paper, the same class of functions
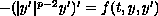 will be given, but with slightly different input data compared to
those from the previous papers, such that the graph
will be given, but with slightly different input data compared to
those from the previous papers, such that the graph
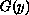 of each solution
of each solution
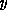 is a rectifiable curve in
is a rectifiable curve in
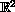 . Moreover,
there is a positive constant which does not depend on
. Moreover,
there is a positive constant which does not depend on
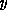 so that
so that
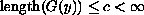 .
.
Submitted November 11, 2004. Published April 24, 2005.
Math Subject Classifications: 35J60, 34B15, 28A75.
Key Words: Nonlinear p-Laplacian; bounded continuous solutions;
graph; qualitative properties; length; rectifiability.
Show me the
PDF file (235K),
TEX file, and other files for this article.
 |
Mervan Pasic
Department of Mathematics
Faculty of Electrical Engineering and Computing
University of Zagreb
Unska 3, 10000 Zagreb, Croatia
email: mervan.pasic@fer.hr |
|---|
Return to the EJDE web page
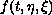 rapidly sign-changing
near the boundary point
rapidly sign-changing
near the boundary point
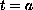 ,
has been constructed so that the
equation
,
has been constructed so that the
equation
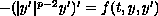 in
in
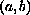 admits continuous
bounded solutions
admits continuous
bounded solutions
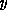 whose graphs
whose graphs
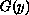 do not possess a finite
length. In this paper, the same class of functions
do not possess a finite
length. In this paper, the same class of functions
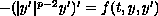 will be given, but with slightly different input data compared to
those from the previous papers, such that the graph
will be given, but with slightly different input data compared to
those from the previous papers, such that the graph
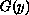 of each solution
of each solution
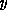 is a rectifiable curve in
is a rectifiable curve in
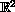 . Moreover,
there is a positive constant which does not depend on
. Moreover,
there is a positive constant which does not depend on
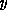 so that
so that
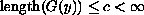 .
.
