Electron. J. Diff. Eqns., Vol. 2005(2005), No. 92, pp. 1-26.

Statistical mechanics of the $N$-point vortex system with random intensities on $\mathbb{R}^2$

Cassio Neri

Abstract:
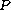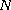The system of N -point vortices on $\mathbb{R}^2$ is considered under the hypothesis that vortex intensities are independent and identically distributed random variables with respect to a law $P$ supported on $(0,1]$. It is shown that, in the limit as $N$ approaches $\infty$, the 1-vortex distribution is a minimizer of the free energy functional and is associated to (some) solutions of the following non-linear Poisson Equation:
$$ -\Delta u(x) = C^{-1}\int_{(0,1]} r\hbox{e}^{-\beta ru(x)- \gamma r|x|^2}P(\hbox{d}r), \quad\forall x\in \mathbb{R}^2, $$
where $\displaystyle C = \int_{(0,1]}\int_{\mathbb{R}^2}\hbox{e}^{-\beta ru(y) - \gamma r|y|^2}\hbox{d} yP(\hbox{d}r)$.

Submitted March 3, 2005. Published August 24, 2005.
Math Subject Classifications: 76F55, 82B5.
Key Words: Statistical mechanics; N-point vortex system; Onsager theory; mean field equation.

Show me the PDF file (328K), TEX file, and other files for this article.

Cassio Neri
Instituto de Matemática
Universidade Federal do Rio de Janeiro
Caixa Postal 68530, CEP: 21945-970, Rio de Janeiro, Brazil
email: cassio@labma.ufrj.br

Return to the EJDE web page