Electron. J. Diff. Eqns.,
Vol. 2006(2006), No. 151, pp. 1-6.
A counterexample to an endpoint bilinear Strichartz inequality
Terence Tao
Abstract:
The endpoint Strichartz estimate
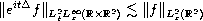
is known to be false by the work of Montgomery-Smith
[2], despite being only "logarithmically
far" from being true in some sense. In this short note we show
that (in sharp contrast to the
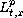 Strichartz estimates) the situation is not improved by passing
to a bilinear setting; more precisely, if
Strichartz estimates) the situation is not improved by passing
to a bilinear setting; more precisely, if
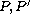 are non-trivial
smooth Fourier cutoff multipliers then we show that
the bilinear estimate
are non-trivial
smooth Fourier cutoff multipliers then we show that
the bilinear estimate
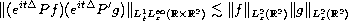
fails even when
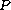 ,
,
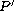 have widely separated supports.
have widely separated supports.
Submitted September 29, 2006. Published December 5, 2006.
Math Subject Classifications: 35J10.
Key Words: Strichartz inequality.
Show me the
PDF file (206K),
TEX file, and other files for this article.
 |
Terence Tao
Department of Mathematics
University of California
Los Angeles, CA 90095-1555, USA
email: tao@math.ucla.edu |
|---|
Return to the EJDE web page
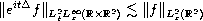
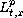 Strichartz estimates) the situation is not improved by passing
to a bilinear setting; more precisely, if
Strichartz estimates) the situation is not improved by passing
to a bilinear setting; more precisely, if
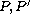 are non-trivial
smooth Fourier cutoff multipliers then we show that
the bilinear estimate
are non-trivial
smooth Fourier cutoff multipliers then we show that
the bilinear estimate
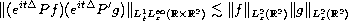
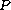 ,
,
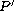 have widely separated supports.
have widely separated supports.
