We derive rigorously homogenized models for the displacement of one compressible miscible fluid by another in fractured porous media. We denote by
 the characteristic size of the heterogeneity
in the medium. A parameter
the characteristic size of the heterogeneity
in the medium. A parameter
![$\alpha \in [0,1]$](gifs/ab.gif) characterizes the
cracking degree of the rock.
We carefully define an adapted microscopic model which is scaled by
appropriate powers of
characterizes the
cracking degree of the rock.
We carefully define an adapted microscopic model which is scaled by
appropriate powers of
 .
We then study its limit as
.
We then study its limit as
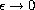 .
Assuming a totally fractured or a partially fractured medium, we obtain
two effective macroscopic limit models.
The first one is a double porosity model. The second one is of single
porosity type but it still contains some effects due to the partial
storage in the matrix part. The convergence is shown using
two-scale convergence techniques.
.
Assuming a totally fractured or a partially fractured medium, we obtain
two effective macroscopic limit models.
The first one is a double porosity model. The second one is of single
porosity type but it still contains some effects due to the partial
storage in the matrix part. The convergence is shown using
two-scale convergence techniques.
