Electron. J. Diff. Eqns.,
Vol. 2008(2008), No. 161, pp. 1-7.
Asymptotic expansion formulas for the maximum of
solutions to diffusive logistic equations
Tetsutaro Shibata
Abstract:
We consider the nonlinear eigenvalue problems
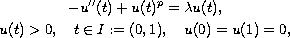
where
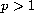 is a constant and
is a constant and
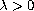 is a parameter.
This equation is well known as the original logistic equation of
population dynamics when
is a parameter.
This equation is well known as the original logistic equation of
population dynamics when
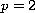 .
We establish the precise asymptotic formula for
.
We establish the precise asymptotic formula for
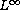 -norm of the solution
-norm of the solution
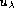 as
as
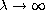 when
when
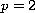 and
and
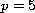 .
.
Submitted May 07, 2008. Published December 9, 2008.
Math Subject Classifications: 34B15.
Key Words: Logistic equation;
 -norm of solutions.
-norm of solutions.
Show me the
PDF file (197 KB),
TEX file, and other files for this article.
 |
Tetsutaro Shibata
Department of Applied Mathematics
Graduate School of Engineering, Hiroshima University
Higashi-Hiroshima, 739-8527, Japan
email: shibata@amath.hiroshima-u.ac.jp
|
|---|
Return to the EJDE web page
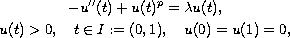
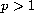 is a constant and
is a constant and
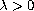 is a parameter.
This equation is well known as the original logistic equation of
population dynamics when
is a parameter.
This equation is well known as the original logistic equation of
population dynamics when
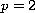 .
We establish the precise asymptotic formula for
.
We establish the precise asymptotic formula for
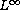 -norm of the solution
-norm of the solution
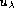 as
as
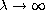 when
when
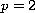 and
and
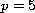 .
.
