Electron. J. Diff. Eqns., Vol. 2008(2008), No. 33, pp. 1-14.

A nonhomogeneous backward heat problem: Regularization and error estimates

Dang Duc Trong, Nguyen Huy Tuan

Abstract:
We consider the problem of finding the initial temperature, from the final temperature, in the nonhomogeneous heat equation
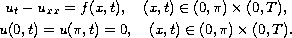
$$\displaylines{ u_t-u_{xx}= f(x,t),\quad (x,t)\in (0,\pi)\times (0,T),\cr u(0,t)= u(\pi,t)= 0, \quad (x,t) \in (0,\pi)\times(0,T). }$$
This problem is known as the backward heat problem and is severely ill-posed. Our goal is to present a simple and convenient regularization method, and sharp error estimates for its approximate solutions. We illustrate our results with a numerical example.

Submitted November 9, 2007. Published March 6, 2008.
Math Subject Classifications: 35K05, 35K99, 47J06, 47H10.
Key Words: Backward heat problem; ill-posed problem; nonhomogeneous heat equation; contraction principle.

Show me the PDF file (255 KB), TEX file, and other files for this article.

Dang Duc Trong
Department of Mathematics and Computer Sciences
Hochiminh City National University
227 Nguyen Van Cu, Hochiminh City, Vietnam
email: ddtrong@mathdep.hcmuns.edu.vn
Nguyen Huy Tuan
Department of Information Technology and Applied Mathematics
Ton Duc Thang University
98 Ngo Tat To, Hochiminh City, Vietnam
email: tuanhuy_bs@yahoo.com

Return to the EJDE web page