Electron. J. Diff. Equ.,
Vol. 2009(2009), No. 148, pp. 1-6.
A remark on the regularity for the 3D Navier-Stokes equations
in terms of the two components of the velocity
Sadek Gala
Abstract:
In this note, we study the regularity of Leray-Hopf weak solutions
to the Navier-Stokes equation, with the condition
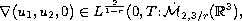
where
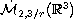 is the
Morrey-Campanato space for
is the
Morrey-Campanato space for
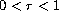 . Since
. Since
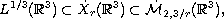
the above regularity condition allows us to improve the results
obtained by Fan and Gao [6].
Submitted November 5, 2009. Published November 25, 2009.
Math Subject Classifications: 35Q30, 35K15, 76D05.
Key Words: Navier-Stokes equations; regularity criterion;
Morrey-Campanato spaces.
Show me the PDF file (209 KB),
TEX file, and other files for this article.
Sadek Gala
Department of Mathematics, University of Mostaganem
Box 227, Mostaganem 27000, Algeria
email: sadek.gala@gmail.com
|
Return to the EJDE web page
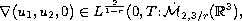
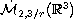 is the
Morrey-Campanato space for
is the
Morrey-Campanato space for
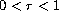 . Since
. Since