This article concerns the oblique derivative problems for second-order quasilinear degenerate equations of mixed type with several characteristic boundaries, which include the Tricomi problem as a special case. First we formulate the problem and obtain estimates of its solutions, then we show the existence of solutions by the successive iterations and the Leray-Schauder theorem. We use a complex analytic method: elliptic complex functions are used in the elliptic domain, and hyperbolic complex functions in the hyperbolic domain, such that second-order equations of mixed type with degenerate curve are reduced to the first order mixed complex equations with singular coefficients. An application of the complex analytic method, solves (1.1) below with
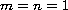 ,
,
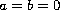 ,
which was posed as
an open problem by Rassias.
,
which was posed as
an open problem by Rassias.
