This article concerns the second-order differential equation with integral boundary conditions
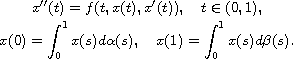
Under the resonance conditions, we construct a projector and then applying coincidence degree theory to establish the existence of solutions.
Yujun Cui
Abstract:
This article concerns the second-order differential
equation with integral boundary conditions
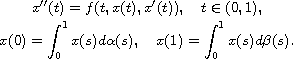
Under the resonance conditions, we construct a projector and then
applying coincidence degree theory to establish the existence of solutions.
Submitted December 26, 2011. Published March 20, 2012.
Math Subject Classifications: 34B15.
Key Words: Coincidence degree; integral boundary-value problem; resonance.
An addendum was attached on March 30, 2012. It corrects several mistakes and provides additional references. See page 10 of this article.
Show me the PDF file (207 KB), TEX file for this article.
 |
Yujun Cui Institute of Mathematics Shandong University of Science and Technology Qingdao 266590, China email: cyj720201@163.com |
|---|
Return to the EJDE web page