Electron. J. Diff. Equ.,
Vol. 2013 (2013), No. 108, pp. 1-10.
Symmetry and regularity of an optimization problem related
to a nonlinear BVP
Claudia Anedda, Fabrizio Cuccu
Abstract:
We consider the functional
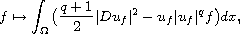
where
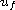 is the unique nontrivial weak solution of the boundary-value
problem
is the unique nontrivial weak solution of the boundary-value
problem
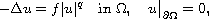
where
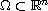 is a bounded smooth domain.
We prove a result of Steiner symmetry preservation and, if
is a bounded smooth domain.
We prove a result of Steiner symmetry preservation and, if
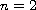 ,
we show the regularity of the level sets of minimizers.
,
we show the regularity of the level sets of minimizers.
Submitted January 7, 2013. Published April 29, 2013.
Math Subject Classifications: 35J20, 35J60, 40K20.
Key Words: Laplacian; optimization problem; rearrangements;
Steiner symmetry; regularity.
Show me the PDF file (250 KB),
TEX file for this article.
 |
Claudia Anedda
Dipartimento di Matematica e Informatica
Universitá di Cagliari
Via Ospedale 72, 09124 Cagliari, Italy
email: canedda@unica.it
|
|---|
 |
Fabrizio Cuccu
Dipartimento di Matematica e Informatica
Universitá di Cagliari
Via Ospedale 72, 09124 Cagliari, Italy
email: fcuccu@unica.it
|
|---|
Return to the EJDE web page
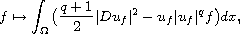
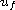 is the unique nontrivial weak solution of the boundary-value
problem
is the unique nontrivial weak solution of the boundary-value
problem
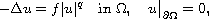
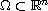 is a bounded smooth domain.
We prove a result of Steiner symmetry preservation and, if
is a bounded smooth domain.
We prove a result of Steiner symmetry preservation and, if
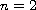 ,
we show the regularity of the level sets of minimizers.
,
we show the regularity of the level sets of minimizers.

