Electron. J. Differential Equations, Vol. 2017 (2017), No. 69, pp. 1-12.

Asymptotic behavior of solutions to a non-autonomous system of two-dimensional differential equations

Songlin Xiao

Abstract:
This article concerns the two-dimensional Bernfeld-Haddock conjecture involving non-autonomous delay differential equations. Employing the differential inequality theory, it is shown that every bounded solution tends to a constant vector as 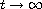$t\to \infty$. Numerical simulations are carried out to verify our theoretical findings.

Submitted February 21, 2017. Published March 10, 2017.
Math Subject Classifications: 34C12, 39A11.
Key Words: Bernfeld-Haddock conjecture; non-autonomous differential equation; time-varying delay; asymptotic behavior.

Show me the PDF file (254 KB), TEX file for this article.

Songlin Xiao
School of Mathematics and Information Science
Guangzhou University
Guangzhou 510006, China
Phone: +8602039366859, Fax: +8602039366859
email: xiaosonglin2017@163.com

Return to the EJDE web page