Electron. J. Differential Equations, Vol. 2018 (2018), No. 02, pp. 1-15.

Global interval bifurcation and convex solutions for the Monge-Ampere equations

Wenguo Shen

Abstract:
In this article, we establish the global bifurcation result from the trivial solutions axis or from infinity for the Monge-Ampere equations with non-differentiable nonlinearity. By applying the above result, we shall determine the interval of $\gamma$, in which there exist radial solutions for the following Monge-Amp\`ere equation
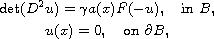
$$\displaylines{ \det(D^2u)= \gamma a(x)F(-u),\quad \text{in } B,\cr u(x)=0,\quad \text{on }\partial B, }$$
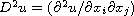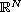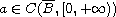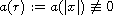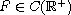where $D^2u=(\partial^2u/\partial x_{i}\partial x_{j})$ is the Hessian matrix of u, where B is the unit open ball of $\mathbb{R}^{N}$, $\gamma$ is a positive parameter. $a\in C(\overline{B}, [0,+\infty))$ is a radially symmetric weighted function and $a(r):= a(|x|)\not\equiv0$ on any subinterval of [0, 1] and the nonlinear term $F\in C(\mathbb{R}^+)$ but is not necessarily differentiable at the origin and infinity. We use global interval bifurcation techniques to prove our main results.

Submitted June 14, 2017. Published January 2, 2018.
Math Subject Classifications: 34B15, 34C10, 34C23.
Key Words: Global bifurcation; interval bifurcation; convex solutions; Monge-Ampere equations.

Show me the PDF file (295 KB), TEX file for this article.

Wenguo Shen
Department of Basic Courses
Lanzhou Institute of Technology
Lanzhou 730050, China
email: shenwg369@163.com

Return to the EJDE web page