In this article, we consider the nonlinear Hartree equation with a sublinear damping and a time-dependent Coulomb potential in
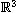 .
We first prove the existence of a global solution
and then obtain the
.
We first prove the existence of a global solution
and then obtain the
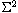 -regularity.
-regularity.
Yanjun Zhao, Binhua Feng
Abstract:
In this article, we consider the nonlinear Hartree equation with
a sublinear damping and a time-dependent Coulomb potential in
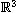 .
We first prove the existence of a global solution
and then obtain the
.
We first prove the existence of a global solution
and then obtain the
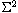 -regularity.
-regularity.
Submitted August 18, 2017. Published September 11, 2018.
Math Subject Classifications: 35J60, 35Q55.
Key Words: Nonlinear Hartree equation; Coulomb potential; sublinear damping.
Show me the PDF file (270 KB), TEX file for this article.
 |
Yanjun Zhao College of Humanities and Sciences Northeast Normal University Changchun 130117, China email: 940508086@qq.com |
|---|---|
 |
Binhua Feng Department of Mathematics Northwest Normal University Lanzhou 730070, China email: binhuaf@163.com |
Return to the EJDE web page