Electron. J. Differential Equations, Vol. 2018 (2018), No. 44, pp. 1-20.

Existence and global behavior of solutions to fractional p-Laplacian parabolic problems

Jacques Giacomoni, Sweta Tiwari

Abstract:
First, we discuss the existence, the uniqueness and the regularity of the weak solution to the following parabolic equation involving the fractional p-Laplacian,
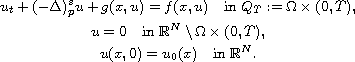
$$\displaylines{ u_t+(-\Delta)_{p}^su +g(x,u)= f(x,u)\quad \text{in } Q_T:=\Omega\times (0,T), \cr u = 0 \quad \text{in } \mathbb{R}^N\setminus\Omega\times(0,T),\cr u(x,0) =u_0(x)\quad \text{in }\mathbb{R}^N. }$$
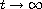Next, we deal with the asymptotic behavior of global weak solutions. Precisely, we prove under additional assumptions on f and g that global solutions converge to the unique stationary solution as $t\to \infty$.

Submitted July 29, 2017. Published February 8, 2018.
Math Subject Classifications: 35K59, 35K55, 35B40.
Key Words: p-fractional operator; existence and regularity of weak solutions; asymptotic behavior of global solutions; stabilization.

Show me the PDF file (309 KB), TEX file for this article.

Jacques Giacomoni
Université de Pau et des Pays de l'Adour
CNRS, LMAP (UMR 5142), Bat. Ipra
avenue de l'Université, Pau, France
email: jacques.giacomoni@univ-pau.fr
Sweta Tiwari
Department of Mathematics
IIT Guwahati, India
email: swetatiwari@iitg.ernet.in

Return to the EJDE web page