Electron. J. Differential Equations,
Vol. 2019 (2019), No. 19, pp. 1-16.
Approximate controllability of Euler-Bernoulli viscoelastic systems
Zhifeng Yang, Zhaosheng Feng
Abstract:
In this article, we study an Euler-Bernoulli viscoelastic control system
which is dissipative due to the presence of the viscoelastic term.
The main feature which distinguishes this paper from other related works
lies in the fact that we no longer impose traditional conditions such as
complete monotonicity and decay property on the kernel function g.
Without loss of generality, we study the system in the case of
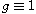 .
By means of the duality principle and the Hahn-Banach theorem, we show that
the system with g=1 is approximately controllable in the appropriate
function space.
.
By means of the duality principle and the Hahn-Banach theorem, we show that
the system with g=1 is approximately controllable in the appropriate
function space.
Submitted February 28, 2018. Published January 30, 2019.
Math Subject Classifications: 93B05, 93C20, 35Q93.
Key Words: Euler-Bernoulli viscoelastic system; approximate controllability;
duality principle; Hahn-Banach theorem.
Show me the PDF file (254 KB),
TEX file for this article.
 |
Zhifeng Yang
College of Mathematics and Statistics
Hengyang Normal University
Hengyang, Hunan 421002, China
email: yzfmath@hynu.edu.cn
|
|---|
 |
Zhaosheng Feng
Department of Mathematics
University of Texas Rio Grande Valley
Edinburg, TX 78539, USA
email: zhaosheng.feng@utrgv.edu
|
|---|
Return to the EJDE web page
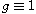 .
By means of the duality principle and the Hahn-Banach theorem, we show that
the system with g=1 is approximately controllable in the appropriate
function space.
.
By means of the duality principle and the Hahn-Banach theorem, we show that
the system with g=1 is approximately controllable in the appropriate
function space.

