Let
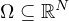 be a bounded domain.
In this article, we investigate the existence of entropy solutions to
the nonlinear elliptic problem
be a bounded domain.
In this article, we investigate the existence of entropy solutions to
the nonlinear elliptic problem
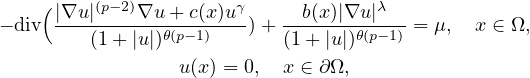
where
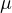 is a diffuse measure with bounded variation on
is a diffuse measure with bounded variation on
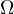 ,
,
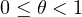 is a positive constants, 1
is a positive constants, 1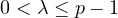 ,
c(x) and b(x) belong to appropriate Lorentz spaces.
,
c(x) and b(x) belong to appropriate Lorentz spaces.
Submitted August 4, 2017. Published August 5, 2019.
Math Subject Classifications: 35R06, 35J70.
Key Words: Entropy solution; noncoercive; nonlinear elliptic equations;
measure data.
Show me the PDF file (419 KB), TEX file for this article.
 |
Shuibo Huang School of Mathematics and Computer Northwest Minzu University Lanzhou, Gansu 730000, China email: huangshuibo2008@163.com |
|---|---|
 |
Tong Su School of Mathematics and Computer Northwest Minzu University Lanzhou, Gansu 730000, China email: 309372469@qq.com |
 |
Xinsheng Du School of Mathematical Sciences Qufu Normal University Qufu, Shandong 273165, China email: duxinsheng1977@163.com |
 |
Xinqiu Zhang School of Mathematics and Statistics Qingdao University Qingdao 266071, China email: xinqiuzhang0220@163.com |
Return to the EJDE web page