Paul W. Eloe, Jeffrey T. Neugebauer
Abstract:
We apply a recent Avery et al. fixed point theorem to the Hammerstein
integral equation
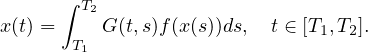
Under certain conditions on G, we show the existence of positive
and positive symmetric solutions. Examples are given where G is a
convolution kernel and where G is a Green's function associated with
different boundary-value problem.
Submitted November 28, 2018. Published August 13, 2019.
Math Subject Classifications: 47H10, 34A08, 34B15, 34B27, 45G10.
Key Words: Hammerstein integral equation; boundary-value problem;
fractional boundary-value problem.
Show me the PDF file (370 KB), TEX file for this article.
 |
Paul W. Eloe Department of Mathematics University of Dayton Dayton, OH 45469, USA email: peloe1@udayton.edu |
|---|---|
 |
Jeffrey T. Neugebauer Department of Mathematics and Statistics Eastern Kentucky University Richmond, KY 40475, USA email: Jeffrey.Neugebauer@eku.edu |
Return to the EJDE web page