Electron. J. Differential Equations,
Vol. 2020 (2020), No. 06, pp. 1-18.
Stationary quantum Zakharov systems involving a higher competing perturbation
Shuai Yao, Juntao Sun, Tsung-Fang Wu
Abstract:
We consider the stationary quantum Zakharov system with a higher
competing perturbation

where
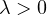 ,
,
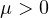 ,
,
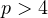 and functions
and functions
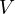 and
and
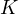 are both
nonnegative. Such problem can not be studied via the common arguments in
variational methods, since Palais-Smale sequences may not be bounded.
Using a constraint approach proposed by us recently, we prove the existence,
multiplicity and concentration of nontrivial solutions for the above problem.
are both
nonnegative. Such problem can not be studied via the common arguments in
variational methods, since Palais-Smale sequences may not be bounded.
Using a constraint approach proposed by us recently, we prove the existence,
multiplicity and concentration of nontrivial solutions for the above problem.
Submitted July 21, 2019. Published January 10, 2020.
Math Subject Classifications: 35J35, 35B38.
Key Words: Quantum Zakharov system; variational methods; multiple solutions.
DOI: 10.58997/ejde.2020.06
Show me the PDF file (380 KB),
TEX file for this article.
 |
Shuai Yao
School of Mathematics and Statistics
Shandong University of Technology
Zibo 255049, China
email: shyao2019@163.com
|
|---|
 |
Juntao Sun
School of Mathematics and Statistics
Shandong University of Technology
Zibo 255049, China
email: jtsun@sdut.edu.cn
|
|---|
 |
Tsung-Fang Wu
Department of Applied Mathematics
National University of Kaohsiung
Kaohsiung 811, Taiwan
email: tfwu@nuk.edu.tw
|
|---|
Return to the EJDE web page

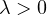 ,
,
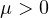 ,
,
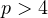 and functions
and functions
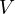 and
and
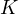 are both
nonnegative. Such problem can not be studied via the common arguments in
variational methods, since Palais-Smale sequences may not be bounded.
Using a constraint approach proposed by us recently, we prove the existence,
multiplicity and concentration of nontrivial solutions for the above problem.
are both
nonnegative. Such problem can not be studied via the common arguments in
variational methods, since Palais-Smale sequences may not be bounded.
Using a constraint approach proposed by us recently, we prove the existence,
multiplicity and concentration of nontrivial solutions for the above problem.


