Electron. J. Differential Equations, Vol. 2020 (2020), No. 117, pp. 1-16.
Existence and nonexistence of radial solutions for
semilinear equations with bounded nonlinearities on exterior domains
Joseph Iaia
Abstract:
In this article we study radial solutions of
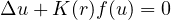 on the exterior of the ball of radius R>0 centered at the origin in
on the exterior of the ball of radius R>0 centered at the origin in
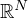 ,
where f is odd with f<0 on
,
where f is odd with f<0 on
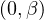 , f>0 on
, f>0 on
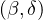 ,
,
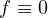 for
for
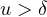 ,
and where the function K(r) is assumed to be positive
and
,
and where the function K(r) is assumed to be positive
and
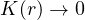 as
as
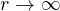 .
The primitive
.
The primitive
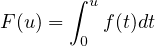 has a ``hilltop'' at
has a ``hilltop'' at
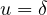 . With mild assumptions on f we prove that if
. With mild assumptions on f we prove that if
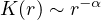 with
with
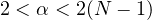 then there are n solutions of
then there are n solutions of
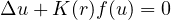 on the exterior of the ball of radius R such that
on the exterior of the ball of radius R such that
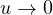 as
as
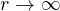 if R>0 is sufficiently small. We also show there are no solutions
if R>0 is sufficiently large.
if R>0 is sufficiently small. We also show there are no solutions
if R>0 is sufficiently large.
Submitted January 6, 2020. Published December 1, 2020.
Math Subject Classifications: 34B40, 35B05.
Key Words: Sublinear equation; radial solution; exterior domain.
DOI: 10.58997/ejde.2020.117
Show me the PDF file (371 KB),
TEX file for this article.
 |
Joseph A. Iaia
Department of Mathematics
University of North Texas, P.O. Box 311430
Denton, TX 76203-5017, USA
email: iaia@unt.edu
|
|---|
Return to the EJDE web page
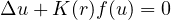 on the exterior of the ball of radius R>0 centered at the origin in
on the exterior of the ball of radius R>0 centered at the origin in
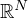 ,
where f is odd with f<0 on
,
where f is odd with f<0 on
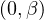 , f>0 on
, f>0 on
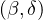 ,
,
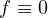 for
for
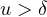 ,
and where the function K(r) is assumed to be positive
and
,
and where the function K(r) is assumed to be positive
and
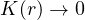 as
as
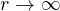 .
The primitive
.
The primitive
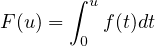 has a ``hilltop'' at
has a ``hilltop'' at
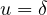 . With mild assumptions on f we prove that if
. With mild assumptions on f we prove that if
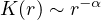 with
with
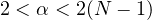 then there are n solutions of
then there are n solutions of
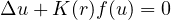 on the exterior of the ball of radius R such that
on the exterior of the ball of radius R such that
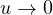 as
as
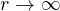 if R>0 is sufficiently small. We also show there are no solutions
if R>0 is sufficiently large.
if R>0 is sufficiently small. We also show there are no solutions
if R>0 is sufficiently large.
