Electron. J. Differential Equations, Vol. 2020 (2020), No. 12, pp. 1-20.
Positive and nodal solutions for nonlinear nonhomogeneous parametric Neumann problems
Nikolaos S. Papageorgiou, Calogero Vetro, Francesca Vetro
Abstract:
We consider a parametric Neumann problem driven by a nonlinear
nonhomogeneous differential operator plus an indefinite potential term.
The reaction term is superlinear but does not satisfy the
Ambrosetti-Rabinowitz condition.
First we prove a bifurcation-type result describing in a precise way the
dependence of the set of positive solutions on the parameter
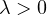 .
We also show the existence of a smallest positive solution. Similar results
hold for the negative solutions and in this case we have a biggest
negative solution. Finally using the extremal constant sign solutions
we produce a smooth nodal solution.
.
We also show the existence of a smallest positive solution. Similar results
hold for the negative solutions and in this case we have a biggest
negative solution. Finally using the extremal constant sign solutions
we produce a smooth nodal solution.
Submitted February 11, 2019. Published January 24, 2020.
Math Subject Classifications: 35J20, 35J60, 58E05.
Key Words: Nonlinear nonhomogeneous differential operator;
nonlinear regularity theory; nonlinear maximum principle;
strong comparison; bifurcation-type theorem; nodal solution;
critical group.
DOI: 10.58997/ejde.2020.12
Show me the PDF file (392 KB),
TEX file for this article.
| |
Nikolaos S. Papageorgiou
National Technical University
Department of Mathematics, Zografou campus
15780, Athens, Greece
email: npapg@math.ntua.gr
|
|---|
 |
Calogero Vetro
University of Palermo
Department of Mathematics and Computer Science
Via Archirafi 34, 90123, Palermo, Italy
email: calogero.vetro@unipa.it
|
|---|
 |
Francesca Vetro
Nonlinear Analysis Research Group
Faculty of Mathematics and Statistics
Ton Duc Thang University
Ho Chi Minh City, Vietnam
email: francescavetro@tdtu.edu.vn
|
|---|
Return to the EJDE web page
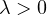 .
We also show the existence of a smallest positive solution. Similar results
hold for the negative solutions and in this case we have a biggest
negative solution. Finally using the extremal constant sign solutions
we produce a smooth nodal solution.
.
We also show the existence of a smallest positive solution. Similar results
hold for the negative solutions and in this case we have a biggest
negative solution. Finally using the extremal constant sign solutions
we produce a smooth nodal solution.

