The Magneto-Hydrodynamic (MHD) system of equations governs viscous fluids subject to a magnetic field and is derived via a coupling of the Navier-Stokes equations and Maxwell's equations. Recently it has become common to study generalizations of fluids-based differential equations. Here we consider the generalized Magneto-Hydrodynamic alpha (gMHD-
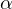 ) system, which differs from the original MHD system by
including an additional non-linear terms (indexed by
) system, which differs from the original MHD system by
including an additional non-linear terms (indexed by
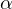 ),
and replacing the Laplace operators by more general Fourier multipliers
with symbols of the form
),
and replacing the Laplace operators by more general Fourier multipliers
with symbols of the form
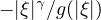 . In [8],
the problem was considered with initial data in the Sobolev space
. In [8],
the problem was considered with initial data in the Sobolev space
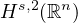 with
with
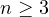 .
Here we consider the problem
with initial data in
.
Here we consider the problem
with initial data in
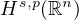 with
with
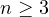 and
and
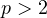 .
Our goal is to minimizing the regularity required for obtaining uniqueness
of a solution.
.
Our goal is to minimizing the regularity required for obtaining uniqueness
of a solution.

