We consider the Cauchy problem for the higher-order nonlinear Schrodinger equation
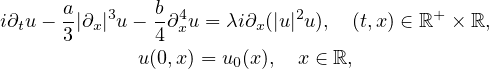
where
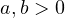 ,
,
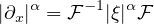 and
and
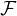 is the Fourier transformation.
Our purpose is to study the large time behavior of the solutions under
the non-zero mass condition
is the Fourier transformation.
Our purpose is to study the large time behavior of the solutions under
the non-zero mass condition
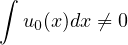 .
.
Pavel I. Naumkin, Isahi Sanchez-Suarez
Abstract:
We consider the Cauchy problem for the higher-order nonlinear Schrodinger equation
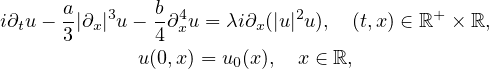
where
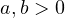 ,
,
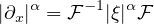 and
and
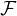 is the Fourier transformation.
Our purpose is to study the large time behavior of the solutions under
the non-zero mass condition
is the Fourier transformation.
Our purpose is to study the large time behavior of the solutions under
the non-zero mass condition
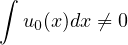 .
.
Submitted June 2, 2018. Published July 22, 2020.
Math Subject Classifications: 35B40, 35Q35.
Key Words: Nonlinear Schrodinger equation; large time asymptotic behavior;
critical nonlinearity; self-similar solutions.
DOI: 10.58997/ejde.2020.77
Show me the PDF file (518 KB), TEX file for this article.
 |
Pavel I. Naumkin Centro de Ciencias Matemáticas UNAM Campus Morelia, AP 61-3 (Xangari) Morelia CP 58089, Michoacán, México email: pavelni@matmor.unam.mx |
|---|---|
| Isahi Sánchez-Suárez Universidad Politécnica de Uruapan CP 60210 Uruapan Michoacán, México email: isahi_ss@hotmail.com |
Return to the EJDE web page