Electron. J. Differential Equations, Vol. 2021 (2021), No. 100, pp. 1-24.
Existence results for nonlinear Schrodinger equations involving the
fractional (p,q)-Laplacian and critical nonlinearities
Huilin Lv, Shenzhou Zheng, Zhaosheng Feng
Abstract:
In this article, we consider the existence of ground state positive solutions for
nonlinear Schrodinger equations of the fractional (p,q)-Laplacian with
Rabinowitz potentials defined in Rn,
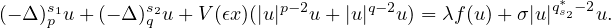
We prove existence by confining different ranges of the parameter λ under the
subcritical or critical nonlinearities caused by σ=0 or 1, respectively.
In particular, a delicate calculation for the critical growth is provided so as to
avoid the failure of a global Palais-Smale condition for the energy functional.
Submitted May 18, 2021. Published December 20, 2021.
Math Subject Classifications: 35R11, 35A15, 58E05.
Key Words: Nonlinear Schrodinger equations; nonlocal (p,q)-Laplacian;
critical growth; Rabinowitz potentials; Nehari manifold.
DOI: https://doi.org/10.58997/ejde.2021.100
Show me the PDF file (422 KB),
TEX file for this article.
 |
Huilin Lv
Department of Mathematics
Beijing Jiaotong University
Beijing 100044, China
email: huilinlv@bjtu.edu.cn
|
|---|
 |
Shenzhou Zheng
Department of Mathematics
Beijing Jiaotong University
Beijing 100044, China
email: shzhzheng@bjtu.edu.cn
|
|---|
 |
Zhaosheng Feng
School of Mathematical and Statistical Sciences
University of Texas Rio Grande Valley
Edinburg, TX 78539, USA
email: zhaosheng.feng@utrgv.edu
|
|---|
Return to the EJDE web page