Electron. J. Differential Equations, Vol. 2021 (2021), No. 38, pp. 1-14.
Optimization problems and mathematical analysis of optimal values in Orlicz spaces
Zahra Donyari, Mohsen Zivari-Rezapour, Behrouz Emamizadeh
Abstract:
This article concerns a minimization problem related to an elliptic equation
in Orlicz-Sobolev spaces. We prove existence and uniqueness of optimal solutions and
show that they are monotone and stable. Furthermore, by employing a characterization
of the tangent cones in
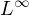 spaces, we derive some qualitative properties
of the optimal solutions. We also derive some results regarding the optimal values.
spaces, we derive some qualitative properties
of the optimal solutions. We also derive some results regarding the optimal values.
Submitted April 12, 2021. Published May 6, 2021.
Math Subject Classifications: 35J25, 49K20.
Key Words: Existence; uniqueness; Orlicz spaces; minimization; tangent cone;
optimal solutions; optimal values.
DOI: https://doi.org/10.58997/ejde.2021.38
Show me the PDF file (354 KB),
TEX file for this article.
 |
Zahra Donyari
Department of Mathematics
Faculty of Mathematical Sciences and Computer
Shahid Chamran University of Ahvaz
Ahvaz, Iran
email: z-donyari@stu.scu.ac.ir
|
|---|
 |
Mohsen Zivari-Rezapour
Department of Mathematics
Faculty of Mathematical Sciences and Computer
Shahid Chamran University of Ahvaz
Ahvaz, Iran
email: mzivari@scu.ac.ir
|
|---|
 |
Behrouz Emamizadeh
School of Mathematical Sciences
University of Nottingham Ningbo China
199 Taikang East Road
Ningbo 315100, China
email: Behrouz.Emamizadeh@nottingham.edu.cn
|
|---|
Return to the EJDE web page
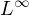 spaces, we derive some qualitative properties
of the optimal solutions. We also derive some results regarding the optimal values.
spaces, we derive some qualitative properties
of the optimal solutions. We also derive some results regarding the optimal values.


