Electron. J. Differential Equations, Vol. 2021 (2021), No. 47, pp. 1-20.
Existence and asymptotic behavior of positive least energy solutions for coupled
nonlinear Choquard equations
Song You, Peihao Zhao, Qingxuan Wang
Abstract:
In this article, we study the coupled nonlinear Schrödinger equations with
Choquard type nonlinearities
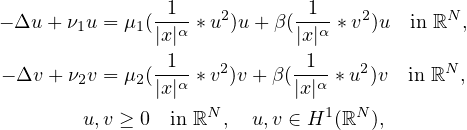
where ν1,ν2,μ1,μ2
are positive constants, β>0
is a coupling constant, N≥3, α in (0,N) ∩ (0,4),
and "*'" is the convolution operator.
We show that the nonlocal elliptic system
has a positive least energy solution for positive small β and positive large
β via variational methods. For the case in which ν1=ν2, μ1≄μ2,
N=3,4,5 and α=N-2, we prove the uniqueness of positive least energy solutions.
Moreover, the asymptotic behaviors of the positive least energy solutions as
β→ 0+ are studied.
Submitted July 17, 2019. Published May 28, 2021.
Math Subject Classifications: 35B40, 35J47, 35J50.
Key Words: Coupled Choquard equations; positive least energy solution;
asymptotic behavior; variational method.
DOI: https://doi.org/10.58997/ejde.2021.47
Show me the PDF file (399 KB),
TEX file for this article.
| |
Song You
Department of Mathematical Sciences
Tsinghua University
Beijing 100084, China
email: yousong@mail.tsinghua.edu.cn
|
|---|
 |
Peihao Zhao
School of Mathematics and Statistics
Lanzhou University
Lanzhou, Gansu 730000, China
email: zhaoph@lzu.edu.cn
|
|---|
| |
Qingxuan Wang
Department of Mathematics
Zhejiang Normal University
Jinhua, Zhejiang 321004, China
email: wangqx@zjnu.edu.cn
|
|---|
Return to the EJDE web page