Electron. J. Differential Equations, Vol. 2021 (2021), No. 80, pp. 1-22.
A fractional Gronwall inequality and the asymptotic behaviour of global
solutions of Caputo fractional problems
Jeffrey R. L. Webb
Abstract:
We study the asymptotic behaviour of global solutions of some nonlinear integral equations
related to some Caputo fractional initial value problems.
We consider problems of fractional order between 0 and 1 and of order between 1 and 2,
each in two cases: when the nonlinearity depends only on the function, and when the
nonlinearity also depends on fractional derivatives of lower order.
Our main tool is a new Gronwall inequality for integrals with singular kernels,
which we prove here, and a related boundedness property of a fractional integral of
an
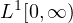 function.
function.
Submitted July 23, 2021. Published September 20, 2021.
Math Subject Classifications: 34A08, 34A12, 26A33, 26D10.
Key Words: Fractional derivatives; asymptotic behaviour; Gronwall inequality;
weakly singular kernel.
DOI: https://doi.org/10.58997/ejde.2021.80
Show me the PDF file (384 KB),
TEX file for this article.
 |
Jeffrey R. L. Webb
School of Mathematics and Statistics
University of Glasgow
Glasgow G12 8SQ, UK
email: jeffrey.webb@glasgow.ac.uk
|
|---|
Return to the EJDE web page
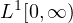 function.
function.
