Electron. J. Differential Equations, Vol. 2022 (2022), No. 55, pp. 1-10.
Double phase equations with an indefinite concave term
Zhenhai Liu, Nikolaos S. Papageorgiou
Abstract:
We consider a Dirichlet problem having a double phase differential
operator with unbalanced growth and reaction involving the combined
effects of a concave (sublinear) and of a convex (superlinear) terms.
We allow the coefficient
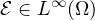 of the concave
term to be sign changing. We show that when
of the concave
term to be sign changing. We show that when
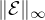 is small the problem has at least two bounded positive solutions.
is small the problem has at least two bounded positive solutions.
Submitted January 12, 2022. Published July 28, 2022.
Math Subject Classifications: 35J75, 35J20, 35J60.
Key Words: Unbalanced growth; generalized Orlicz spaces; positive solution;
concave-convex problem; mountain pass theorem.
Show me the PDF file (333 KB),
TEX file for this article.
DOI: https://doi.org/10.58997/ejde.2022.55
 |
Zhenhai Liu
Guangxi Colleges and Universities
Key Laboratory of Complex System Optimization and Big Data Processing
Yulin Normal University
Yulin 537000, China
email: zhhliu@hotmail.com
|
|---|
| |
Nikolaos S. Papageorgiou
Department of Mathematics
National Technical University
Zografou Campus, 15780 Athens, Greece
email: npapg@math.ntua.gr
|
|---|
Return to the EJDE web page
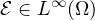 of the concave
term to be sign changing. We show that when
of the concave
term to be sign changing. We show that when
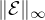 is small the problem has at least two bounded positive solutions.
is small the problem has at least two bounded positive solutions.
