Electron. J. Differential Equations, Vol. 2022 (2022), No. 77, pp. 1-15.
Kirchhoff systems involving fractional
p-Laplacian and singular nonlinearity
Mouna Kratou
Abstract:
In this work we consider the fractional Kirchhoff equations
with singular nonlinearity,
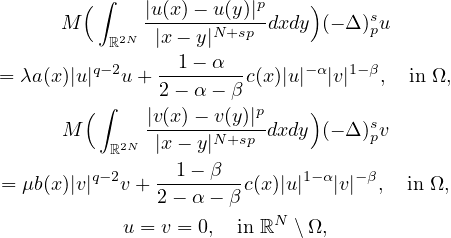
where Ω is a bounded domain in RN with smooth boundary,
N> ps, s in (0,1), 0<α<1, 0< β< 1,
2-α-β<p≤ pθ<q<p*s,
p*s=Np/(N-sp) is the fractional Sobolev exponent,
λ, μ are two parameters, a, b, c in C(overlineΩ)
are non-negative weight functions,
M(t)=k+ltθ-1 with k>0, l,θ≥1, and
(-Δ)sp is the fractional p-laplacian operator.
We prove the existence of multiple non-negative solutions by
studying the nature of the Nehari manifold with respect to the parameters
λ and μ.
Submitted September 11, 2022. Published November 21, 2022.
Math Subject Classifications: 34B15, 37C25, 35R20.
Key Words: Kirchhoff-type equations; fractional p-Laplace operator;
Nehari manifold; singular elliptic system; multiple positive solutions,
DOI: https://doi.org/10.58997/ejde.2022.77
Show me the PDF file (387 KB),
TEX file for this article.
Mouna Kratou
College of sciences at Dammam
University of Imam Abdulrahman Bin Faisal
31441 Dammam, Saudi Arabia
email: mmkratou@iau.edu.sa
|
Return to the EJDE web page