Differential Equations and Computational Simulations III
Electron. J. Diff. Eqns., Conf. 01, 1997, pp. 97-108.
On properties of nonlinear second order systems under
nonlinear impulse perturbations
John R. Graef & Janos Karsai
Abstract:
In this paper, we consider the impulsive second order system
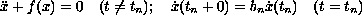
where
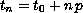
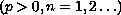 .
In a previous paper, the authors proved that if
.
In a previous paper, the authors proved that if
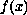 is strictly nonlinear, then this system has
infinitely many periodic solutions.
The impulses account for the main differences in the attractivity properties
of the zero solution. Here, we prove that these periodic
solutions are attractive in some sense, and we give good estimates for
the attractivity region.
is strictly nonlinear, then this system has
infinitely many periodic solutions.
The impulses account for the main differences in the attractivity properties
of the zero solution. Here, we prove that these periodic
solutions are attractive in some sense, and we give good estimates for
the attractivity region.
Published November 12, 1998.
Mathematics Subject Classifications: 34D05, 34D20, 34C15.
Key words and phrases: Asymptotic stability, attractrivity of periodic
solutions, impulsive systems, nonlinear equations, second order systems.
Show me the
PDF file (200K),
TEX file, and other files for this article.
John R. Graef
Department of Mathematics and Statistics,
Mississippi State University
Mississippi State, MS 39762 USA.
Email address: graef@math.msstate.edu
Janos Karsai
Department of Medical Informatics,
Albert Szent-Gyorgyi Medical University
Szeged, Koranyi fasor 9, Hungary
Email address: karsai@silver.szote.u-szeged.hu
Return to the Proceedings of Conferences:
Electr. J. Diff. Eqns.
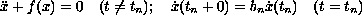
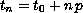
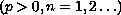 .
In a previous paper, the authors proved that if
.
In a previous paper, the authors proved that if
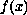 is strictly nonlinear, then this system has
infinitely many periodic solutions.
The impulses account for the main differences in the attractivity properties
of the zero solution. Here, we prove that these periodic
solutions are attractive in some sense, and we give good estimates for
the attractivity region.
is strictly nonlinear, then this system has
infinitely many periodic solutions.
The impulses account for the main differences in the attractivity properties
of the zero solution. Here, we prove that these periodic
solutions are attractive in some sense, and we give good estimates for
the attractivity region.