2001-Luminy conference on Quasilinear Elliptic and Parabolic Equations
and Systems
Electron. J. Diff. Eqns., Conf. 08, 2002, pp. 53-83.
Sets of admissible initial data for porous-medium
equations with absorptions
Emmanuel Chasseigne & Juan Luis Vazquez
Abstract:
In this article, we study a porous-medium equation with absorption
in
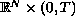 or in
or in
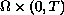 :
:
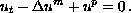
We give a rather complete qualitative picture of the initial
trace problem in all the range
 ,
,
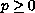 .
We consider nonnegative Borel measures as initial data
(not necessarily locally bounded)
and discuss whether or not the Cauchy problem admits a solution.
In the case of non-admissible data we prove the existence of some
projection operators which map any Borel measure to an admissible
measure for this equation.
.
We consider nonnegative Borel measures as initial data
(not necessarily locally bounded)
and discuss whether or not the Cauchy problem admits a solution.
In the case of non-admissible data we prove the existence of some
projection operators which map any Borel measure to an admissible
measure for this equation.
Show me the
PDF file (340K),
TEX file, and other files for this article.
 |
Emmanuel Chasseigne
Laboratoire de Mathematiques et Physique Theorique
Universite de Tours
Parc de Grandmont, 37200 Tours, France.
E-mail: echasseigne@univ-tours.fr |
|---|
 |
Juan Luis Vazquez
Departamento de Matematicas
Universidad Autonoma de Madrid
28046 Madrid, Spain.
E-mail: juanluis.vazquez@uam.es
|
|---|
Return to the table of contents
for this conference.
Return to the EJDE web page
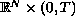 or in
or in
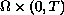 :
:
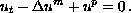
 ,
,
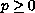 .
We consider nonnegative Borel measures as initial data
(not necessarily locally bounded)
and discuss whether or not the Cauchy problem admits a solution.
In the case of non-admissible data we prove the existence of some
projection operators which map any Borel measure to an admissible
measure for this equation.
.
We consider nonnegative Borel measures as initial data
(not necessarily locally bounded)
and discuss whether or not the Cauchy problem admits a solution.
In the case of non-admissible data we prove the existence of some
projection operators which map any Borel measure to an admissible
measure for this equation.

