 -harmonic Functions
-harmonic Functions
Electron. J. Diff. Eqns., Vol. 1995(1995), No. 04, pp. 1-5.
 -harmonic Functions
-harmonic Functions
Peter Lindqvist & Juan J. Manfredi
Abstract:
The Harnack inequality for nonnegative viscosity
solutions of the equation
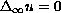 is proved, extending a previous result of L.C. Evans for smooth
solutions. The method of proof consists in considering
is proved, extending a previous result of L.C. Evans for smooth
solutions. The method of proof consists in considering
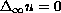 as the limit as
as the limit as
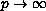 of the more familiar p-harmonic
equation
of the more familiar p-harmonic
equation
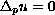 .
.
Submitted February 22, 1995. Published April 3, 1995.
Math Subject Classification: 35J70, 26A16.
Key words: Harnack inequality, p-harmonic equations.
Show me the PDF file (108 KB), TEX file, and other files for this article.
Juan J. Manfredi
Department of Mathematics,
University of Pittsburgh,
Pittsburgh, PA 15260, USA
e-mail: juanjo@na0b.math.pitt.edu