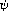 -dichotomy
for homogeneous linear differential equations
-dichotomy
for homogeneous linear differential equations
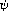 -dichotomy
for homogeneous linear differential equations
-dichotomy
for homogeneous linear differential equations
Pham Ngoc Boi
Abstract:
In this article we present some conditions for the
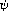 -dichotomy
of the homogeneous linear differential equation
-dichotomy
of the homogeneous linear differential equation
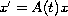 . Under our condition every
. Under our condition every
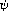 -integrally
bounded function
-integrally
bounded function
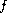 the nonhomogeneous linear differential equation
the nonhomogeneous linear differential equation
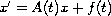 has at least one
has at least one
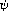 -bounded
solution on
-bounded
solution on
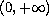 .
.
Submitted December 18, 2005. Published March 26, 2006.
Math Subject Classifications: 34A12, 34C11, 34D05.
Key Words:
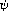 -bounded;
-bounded;
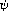 -integrable;
-integrable;
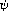 -integrally bounded;
-integrally bounded;
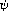 -exponential.
-exponential.
Show me the PDF file (234K), TEX file, and other files for this article.
 |
Pham Ngoc Boi Department of Mathematics Vinh University Vinh City, Vietnam email: pnboi_vn@yahoo.com |
|---|
Return to the EJDE web page