Electron. J. Differential Equations,
Vol. 2017 (2017), No. 26, pp. 1-7.
Inverse problems for Sturm-Liouville operators with boundary
conditions depending on a spectral parameter
Murat Sat
Abstract:
In this article, we study the inverse problem for Sturm-Liouville
operators with boundary conditions dependent on the spectral
parameter. We show that the potential q(x) and coefficient
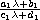 functions can be uniquely
determined from the particular set of eigenvalues.
functions can be uniquely
determined from the particular set of eigenvalues.
Submitted May 5, 2016. Published January 24, 2017.
Math Subject Classifications: 34B05, 34L20, 47E05.
Key Words: Inverse problem; uniqueness theorem; eigenvalue; spectral parameter.
Show me the PDF file (201 KB),
TEX file for this article.
 |
Murat Sat
Department of Mathematics
Faculty of Science and Art
Erzincan University
Erzincan, 24100, Turkey
email: murat_sat24@hotmail.com
|
|---|
Return to the EJDE web page
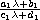 functions can be uniquely
determined from the particular set of eigenvalues.
functions can be uniquely
determined from the particular set of eigenvalues.
