Electron. J. Differential Equations, Vol. 2020 (2020), No. 112, pp. 1-29.
Pyramidal traveling fronts in the Belousov-Zhabotinskii reaction-diffusion
systems in R^3
Luyi Ma, Hong-Tao Niu, Zhi-Cheng Wang
Abstract:
In this article, we consider a diffusion system with the Belousov-Zhabotinskii
(BZ for short) chemical reaction. The existence and stability of V-shaped
traveling fronts for the BZ system in
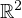 had been proved in
our previous papers [30, 31].
Here we establish the existence and stability of pyramidal traveling fronts
for the BZ system in
had been proved in
our previous papers [30, 31].
Here we establish the existence and stability of pyramidal traveling fronts
for the BZ system in
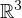 .
.
Submitted April 3, 2020. Published November 12, 2020.
Math Subject Classifications: 35K40, 35K57, 35C07.
Key Words: Belousov-Zhabotinskii reaction; pyramidal traveling fronts;
supersolution; subsolution.
DOI: 10.58997/ejde.2020.112
Show me the PDF file (504 KB),
TEX file for this article.
 |
Luyi Ma
School of Mathematics and Statistics
Lanzhou University
Lanzhou, Gansu 730000, China
email: maly16@lzu.edu.cn
|
|---|
 |
Hong-Tao Niu
School of Mathematics and Statistics
Xuzhou Institute of Technology
Xuzhou, Jiangsu 221000, China
email: aniuht@163.com
|
|---|
 |
Zhi-Cheng Wang
School of Mathematics and Statistics
Lanzhou University
Lanzhou, Gansu 730000, China
email: wangzhch@lzu.edu.cn
|
|---|
Return to the EJDE web page
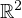 had been proved in
our previous papers [30, 31].
Here we establish the existence and stability of pyramidal traveling fronts
for the BZ system in
had been proved in
our previous papers [30, 31].
Here we establish the existence and stability of pyramidal traveling fronts
for the BZ system in
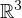 .
.


