Electron. J. Differential Equations, Vol. 2021 (2021), No. 10, pp. 1-12.
Complete classification of bifurcation curves for a multiparameter diffusive logistic
problem with generalized Holling type-IV functional response
Jyun-Yuan Ciou, Tzung-Shin Yeh
Abstract:
We study exact multiplicity and bifurcation curves of positive solutions for
the diffusive logistic problem with generalized Holling type-IV functional
response
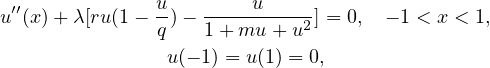
where the quantity in brackets is the growth rate function
and
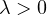 is a bifurcation parameter. On the
is a bifurcation parameter. On the
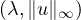 -plane, we give a complete classification of
two qualitatively different bifurcation curves: a C-shaped curve
and a monotone increasing curve.
-plane, we give a complete classification of
two qualitatively different bifurcation curves: a C-shaped curve
and a monotone increasing curve.
Submitted April 5, 2019. Published February 23, 2021.
Math Subject Classifications: 34B15, 34B18.
Key Words: Bifurcation curve; exact multiplicity; diffusive logistic problem;
Holling type-IV functional response; time map.
DOI: https://doi.org/10.58997/ejde.2021.10
Show me the PDF file (783 KB),
TEX file for this article.
 |
Jyun-Yuan Ciou
Department of Applied Mathematics
National University of Tainan
Tainan 700, Taiwan ROC
email: b7626529@gmail.com
|
|---|
 |
Tzung-Shin Yeh
Department of Applied Mathematics
National University of Tainan
Tainan 700, Taiwan, ROC
email: tsyeh@mail.nutn.edu.tw
|
|---|
Return to the EJDE web page
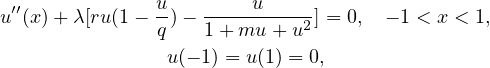
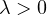 is a bifurcation parameter. On the
is a bifurcation parameter. On the
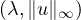 -plane, we give a complete classification of
two qualitatively different bifurcation curves: a C-shaped curve
and a monotone increasing curve.
-plane, we give a complete classification of
two qualitatively different bifurcation curves: a C-shaped curve
and a monotone increasing curve.

