Electron. J. Differential Equations, Vol. 2021 (2021), No. 71, pp. 1-10.
Existence of bounded global solutions for fully parabolic attraction-repulsion
chemotaxis systems with signal-dependent sensitivities and without logistic source
Yutaro Chiyo, Masaaki Mizukami, Tomomi Yokota
Abstract:
This article concerns the parabolic attraction-repulsion
chemotaxis system with signal-dependent sensitivities
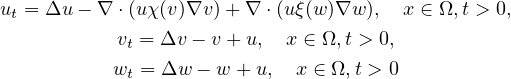
under homogeneous Neumann boundary conditions and initial conditions,
where
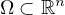
 is a bounded domain with
smooth boundary,
is a bounded domain with
smooth boundary,
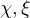 are functions satisfying certain conditions.
Existence of bounded global classical solutions to the system with logistic source
and logistic damping have been obtained in [1].
This article establishes the existence of global bounded classical solutions
with logistic damping.
are functions satisfying certain conditions.
Existence of bounded global classical solutions to the system with logistic source
and logistic damping have been obtained in [1].
This article establishes the existence of global bounded classical solutions
with logistic damping.
Submitted April 8, 2021. Published September 10, 2021.
Math Subject Classifications: 35A01, 35Q92, 92C17.
Key Words: Chemotaxis; attraction-repulsion; existence; boundedness.
DOI: https://doi.org/10.58997/ejde.2021.71
Show me the PDF file (329 KB),
TEX file for this article.
 |
Yutaro Chiyo
Department of Mathematics
Tokyo University of Science
1-3, Kagurazaka, Shinjuku-ku
Tokyo 162-8601, Japan
email: ycnewssz@gmail.com
|
|---|
 |
Masaaki Mizukami
Department of Mathematics
Faculty of Education
Kyoto University of Education
1, Fujinomori, Fukakusa, Fushimi-ku
Kyoto 612-8522, Japan
email: masaaki.mizukami.math@gmail.com
|
|---|
 |
Tomomi Yokota
Department of Mathematics
Tokyo University of Science
1-3, Kagurazaka, Shinjuku-ku
Tokyo 162-8601, Japan
email: yokota@rs.tus.ac.jp
|
|---|
Return to the EJDE web page
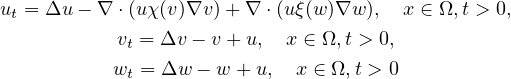
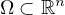
 is a bounded domain with
smooth boundary,
is a bounded domain with
smooth boundary,
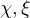 are functions satisfying certain conditions.
Existence of bounded global classical solutions to the system with logistic source
and logistic damping have been obtained in [1].
This article establishes the existence of global bounded classical solutions
with logistic damping.
are functions satisfying certain conditions.
Existence of bounded global classical solutions to the system with logistic source
and logistic damping have been obtained in [1].
This article establishes the existence of global bounded classical solutions
with logistic damping.


